(475d) Ionization and Conformation Consistency in Weak Polyelectrolyte Coacervation

Electrostatic interactions are ubiquitous in biomolecules and are a major determining factor for the conformation of the polypeptides and for the formation of the coacervate (condensate of polypeptides) that is believed to be responsible for the adhesion properties. For example, sandcastle worms produce a glue that is composed of a mixture of three highly polar proteins with two of them containing approximately 15% cationic residues and the third composed of 60-90% anionic residues1. Clearly, the association of these oppositely charged proteins is key to the successful application of the adhesive by forming a coacervate structure that can be dispersed without disintegrating into an aqueous environment. However, the mechanisms by which this coacervation forms and its spontaneous solidification at interfaces remains poorly understood. A key to capturing this phenomenon is a correct treatment of the electrostatic interactions in a polymer, not only between the charged residues and other ions and polymers, but also between residues within its own backbone.

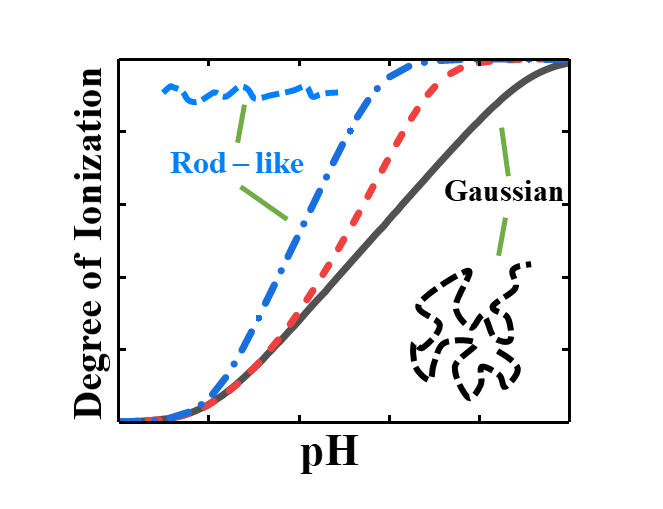
A successful description of the phase behaviour of charged polymers requires a self-consistent treatment of the coupling between electrostatic correlations and chain conformation[1]. Weak polyelectrolytes further complicate this coupling because the ionization of the polymer is also dependent on the inter- and intramolecular correlations and the chain conformation due to the charge regulation of its monomers as shown in Figure 1. Unfortunately, the protonation/deprotonation of monomer in a weak polymer cannot be treated in the same manner as an isolated acid or base due to its correlations with other charged monomers in the same polymer (i.e., the intramolecular interactions influence the ionization of monomers in the same chain). Thus, we must self-consistently treat the segment-level ionization of the polymer, the polymer conformation, and the electrostatic correlations that will depend on the solution conditions since the presence of ions will partially screen the interactions between monomers.

We utilize a liquid-state method to account for the effect of the local ionic environment on both the inter- and intrachain correlations within the framework of thermodynamic perturbation theory. Specifically, we account for the intermolecular correlations resulting from excluded volume and electrostatic interactions through fundamental measure theory[2] and mean spherical approximation (MSA)[3]. The intrachain correlations are described through an approximate form of the radial distribution function from MSA which takes on a form similar to that of the Debye-Huckel screened potential[4]. The liquid-state method is incorporated into a site-binding model for weak polyelectrolytes that accounts for the ionization state of each monomer (viz. charged and uncharged due to its protonation status) in a given polymer chain. However, it becomes computationally infeasible to include non-neighbouring intrachain correlations explicitly in the site-binding model. We take advantage of the Gibbs-Bogoliubov variational principle to account for the important intrachain correlations in a computationally efficient, yet accurate, manner by treating them implicitly based on the solvable reference system (viz. the nearest-neighbour model)[5]. Lastly, a worm-like chain model is introduced to describe the change in conformation of the polymer due to the long-range intrachain correlations[6].

As a demonstration of our new methodology, we first investigate how the solution condition influences the ionization and conformational properties of the polymer. Specifically, we show the importance of intrachain correlations and their resulting effects on the segment-level ionization of the polymer. Next, we investigate phase behaviour of weak polyelectrolytes in a bulk solution. Since our approach automatically accounts for the self-consistent coupling of ionization, conformation, and electrostatic correlations of the polymer, we are able to account for change in ionization and conformation of the polymer due to the differences in the ion and polymer concentration between the dilute and concentrated phases.

References:

[1] K. Shen and ZG. Wang. Macromolecules. 51. 1706-1717 (2018)

[2] R. Roth, J. Phys.: Condens. Matter, 22 063102 (2010)

[3] R. Triolo, J. Grigera, and L. Blum. J. Phys. Chem., 80, 1858-1861 (1976)

[4] D. Henderson and W.R. Smith, J. Stat. Phys., 19, 191-200 (1978)

[5] A.P. Hughes, U. Thiele, and A.J. Archer, Am. J. Phys., 82, 1119-1129 (2014)

[6] ZG. Wang, Macromolecules, 50, 9073-9114 (2017)