(492i) Effect of Surfactants on Elongated Bubbles in Rectangular Capillary Channels: Liquid Encapsulation and Bursting

The complex dynamics that characterise micro-scale flows are a central topic to diverse applications across different disciplines. These range from electronics in the form of modern two-phase coolers [1] and energy production for oil recovery processes in porous media [2], to biological and medical applications as potential methods for cleaning of bacteria and medical therapy [3]. Microfluidic gas-liquid systems in particular have attracted substantial attention as examples of these are found in a number of naturally-occurring systems, such as obstructed pulmonary airways in respiration [4] and volcanic conduits [5]. One crucial commonality between these applications is the almost ubiquitous presence of surface-active agents, which considerably alter the interacting dynamics between surface tension, viscous, and inertial forces. To name a few relevant examples, surfactants are employed in microfluidic synthesis of nanoparticles for stability and aggregation prevention [6] and have been proposed as a solution to pulmonary airway closures [7].

The prominence of the flow of bubbles in liquid-filled microchannels has led to extensive experimental, theoretical, and computational research over the years. Significant progress has been made on the characterisation of bubble tail undulatory structures [8], as well as on understanding the influence of capillarity on the liquid film and bubble morphology [9]. It has been found both experimentally [10, 11] and numerically [12, 13, 14] that systems with high capillary numbers (Ca > 0.05) in particular develop highly unstable and deformable bubbles that may entrap liquid drops or jets at their tail. These liquid structures may subsequently rupture the bubble or remain within its domain. Despite the potential applications of these liquid-gas structures as alternative methods to produce compound drops [12], understanding of their formation mechanisms and control remains incomplete.

In this study we investigate the morphology of elongated gas bubbles under high Ca and Reynolds (Re) conditions in the presence of surfactants to elucidate and understand the phenomena of encapsulation/rupture in square channels. We perform a comprehensive parametric sweep of several surfactant- and flow-related dimensionless numbers, such as Ca, Peclet number (Pe), Biot number (Bi), Damkohler number (Da), and elasticity number (βs). For this purpose, we employ three-dimensional direct numerical simulations based on a hybrid interface tracking/level-set method proposed in [15]. These simulations consider the presence, transport, and exchange of surfactants in the bulk of liquid and the gas liquid interface. As previously mentioned, a limited number of researchers have highlighted the occurrence of the encapsulation/rupture phenomena. Nonetheless, to the best of our knowledge, the field is still missing a thorough and systematic investigation of the underlying dynamics involved in the process for systems with non-negligible inertia and non-axisymmetric channel cross-sections.

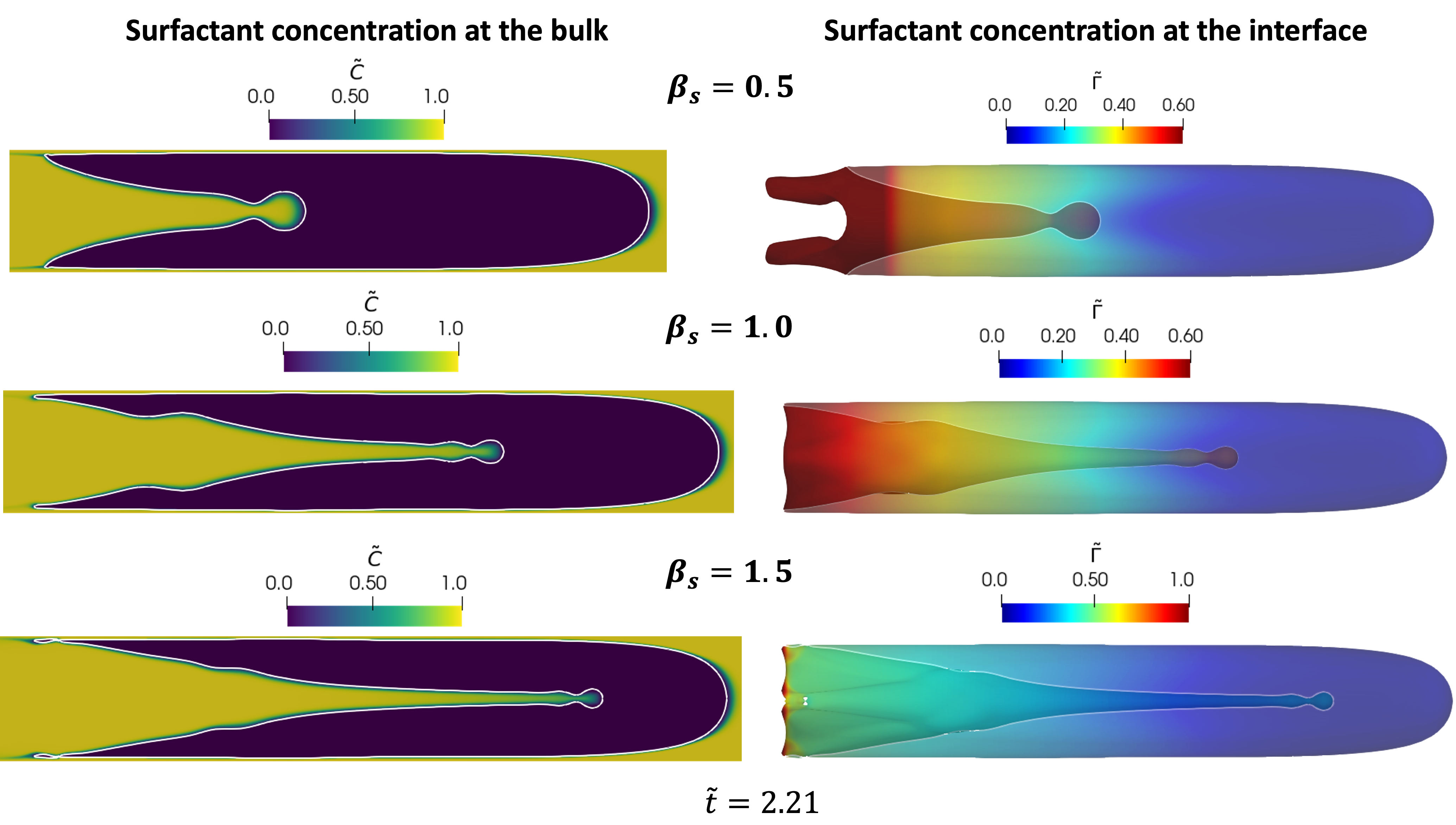
The results of the simulations have led to the identification of different regimes of encapsulation and rupture within the bubble, which are characterised by several proposed parameters, such as the size and velocity of the encapsulated drop, the time of encapsulation, the depth of the liquid jet in the bubble, and the time and location of rupture. It is found that, for typical surfactant properties and operating conditions in the channel, the sets of Pe and Da tested did not have a major influence on the regime of encapsulation obtained. Bi and βs (which quantifies the sensitivity of surface tension to the interface surfactant concentration), on the other hand, significantly alter the liquid-gas structure. Specifically, higher βs are associated with longer bubbles and longer depths of liquid jet penetration at the back of the bubble (see figure attached). In contrast, higher Bi are found to produce larger encapsulated drops as a consequence of increased surfactant desorption rates. The effects of Marangoni stresses on the gas-liquid interface are also highlighted as they are found to induce bubble encapsulation at shorter times than Marangoni-free cases. The characterisation of the different encapsulation/rupture regimes are discussed in the context of potential applications.

References

[1] T. Karayiannis and M. Mahmoud, “Flow boiling in microchannels: Fundamentals and applications,” Applied Thermal Engineering, vol. 115, pp. 1372–1397, 2017. [Online]. Available: https://www.sciencedirect.com/science/ article/pii/S1359431116314090

[2] A. Perazzo, G. Tomaiuolo, V. Preziosi, and S. Guido, “Emulsions in porous media: From single droplet behavior to applications for oil recovery,” Advances in Colloid and Interface Science, vol. 256, pp. 305–325, 2018. [Online]. Available: https://www.sciencedirect.com/science/article/pii/S0001868618300174

[3] Y. Hu, S. Bian, J. Grotberg, M. Filoche, J. White, S. Takayama, and J. B. Grotberg, “A microfluidic model to study fluid dynamics of mucus plug rupture in small lung airways,” Biomicrofluidics, vol. 9, no. 4, p. 044119, 2015. [Online]. Available: https://doi.org/10.1063/1.4928766

[4] D. Halpern, H. Fujioka, S. Takayama, and J. B. Grotberg, “Liquid and surfactant delivery into pulmonary airways,” Respiratory Physiology Neurobiology, vol. 163, no. 1, pp. 222 231, 2008, respiratory Biomechanics. [Online]. Available: https://www.sciencedirect.com/science/article/pii/S1569904808001389

[5] C. R. Carrigan and J. C. Eichelberger, “Zoning of magmas by viscosity in volcanic conduits,” Nature, vol. 343, no. 6255, pp. 248–251, 1991. [Online]. Available: https://www.nature.com/articles/343248a0#citeas

[6] J.-C. Baret, “Surfactants in droplet-based microfluidics,” Lab Chip, vol. 12, pp. 422–433, 2012. [Online]. Available: http://dx.doi.org/10.1039/C1LC20582J

[7] M. Heil, A. L. Hazel, and J. A. Smith, “The mechanics of airway closure,” Respiratory Physiology Neurobiology, vol. 163, no. 1, pp. 214–221, 2008, respiratory Biomechanics. [Online]. Available: https://www.sciencedirect.com/science/article/pii/S1569904808001286

[8] A. Batchvarov, L. Kahouadji, M. Magnini, C. R. Constante-Amores, S. Shin, J. Chergui, D. Juric, R. V. Craster, and O. K. Matar, “Effect of surfactant on elongated bubbles in capillary tubes at high reynolds number,” Phys. Rev. Fluids, vol. 5, p. 093605, Sep 2020. [Online]. Available: https://link.aps.org/doi/10.1103/PhysRevFluids.5.093605

[9] M. Magnini and O. Matar, “Morphology of long gas bubbles propagating in square capillaries,” International Journal of Multiphase Flow, vol. 129, p. 103353, 2020. [Online]. Available: https://www.sciencedirect.com/science/article/pii/S0301932220303360

[10] W. L. Olbricht and D. M. Kung, “The deformation and breakup of liquid drops in low reynolds number flow through a capillary,” Physics of Fluids A: Fluid Dynamics, vol. 4, no. 7, pp. 1347–1354, 1992. [Online]. Available: https://doi.org/10.1063/1.858412

[11] H. Goldsmith and S. Mason, “The flow of suspensions through tubes. ii. single large bubbles,” Journal of Colloid Science, vol. 18, no. 3, pp. 237–261, 1963. [Online]. Available: https://www.sciencedirect.com/science/article/pii/0095852263900151

[12] D. Izbassarov and M. Muradoglu, “A computational study of two-phase viscoelastic systems in a capillary tube with a sudden contraction/expansion,” Physics of Fluids, vol. 28, no. 1, p. 012110, 2016. [Online]. Available: https://doi.org/10.1063/1.4939940

[13] B. Nath, G. Biswas, A. Dalal, and K. C. Sahu, “Migration of a droplet in a cylindrical tube in the creeping flow regime,” Phys. Rev. E, vol. 95, p. 033110, Mar 2017. [Online]. Available: https://link.aps.org/doi/10.1103/PhysRevE.95.033110

[14] O. Atasi, B. Haut, A. Pedrono, B. Scheid, and D. Legendre, “Influence of soluble surfactants and deformation on the dynamics of centered bubbles in cylindrical microchannels,” Langmuir, vol. 34, no. 34, pp. 10 048–10 062, 2018, pMID: 30040422.