(441i) Relation between Defect Dynamics and Rheology in a Sheared Lamellar Mesophase.

Lamellar mesophases typically contain alternating water/surfactant, water/oil/surfactant layers or immiscible segments of a block coopolymer. The equilibrium (minimum energy) phase is one where the layers are aligned with layer normals in the same direction. Under shear, one would expect the layers to align with unit normal velocity gradient direction. In this alignment, the fluid should have viscosity only a few times that of water by the inverse sum rule for the viscosity. However, real lamellar fluids have viscosities that are several orders of magnitudes higher than water. For this reason, lamellar mesophases are used in products which require very high viscosity, such as hair conditioners and butter substitutes. The reason for these high viscosity is a puzzle.

The rheology of lamellar mesophases is complicated due to the two-way coupling between structure and rheology. A perfectly aligned lamellar phase, for example, exhibits fluid like behaviour when the normal to the lamellae is along the shear or vorticity direction, but has a solid like resistance to flow when the normal is along the flow direction. In addition, even though a perfect defect free stack of layers is the final equilibrium state, real samples are rarely defect free due to kinetic constraints. The lamellar spacing is typically small compared to macroscopic scales (the distance between layers in lyotropic liquid crystalline phases is usually a few hundred Angstroms and a macro-scopic sample contains 10⁴ − 10⁶ lamellae), and so a flowing lamellar mesophase cannot be modeled using a microscopic description. It is necessary to use different simulation techniques (molecular, mesoscale, macroscale) for accurately capturing the rheology of lamellar phases.

A multiscale modeling methodology has been developed to link molecular and mesoscale simulations. Here, the objective is to examine the relation between the defect dynamics and the rheology in a mesoscale model based on a concentration field (phase field) description. The mesoscale model is based on an order parameter which distinguishes between the hydrophilic and hydrophobic constituents in a lamellar mesophase. The order parameter field is described by a Landau-Ginzburg free energy functional with an additional bending term that results in a sinusoidal modulation of the concentration field at equilibrium. The equation for the order parameter is coupled with the fluid mass and momentum equations to study the evolution of the lamellar structure under shear flow. The simulations are carried out using the `Lattice Boltzmann' (LBM) technique, in conjunction with a two-population model for treating the fluid and the order parameter field. The simulations are performed at low Reynolds number based on the strain rate and the cross-stream length scale, and the two other dimensionless parameters are the Schmidt number which is a dimensionless ratio of order parameter and momentum diffusivity and a dimensionless ratio of the bending stiffness and the viscous stress in the fluid.

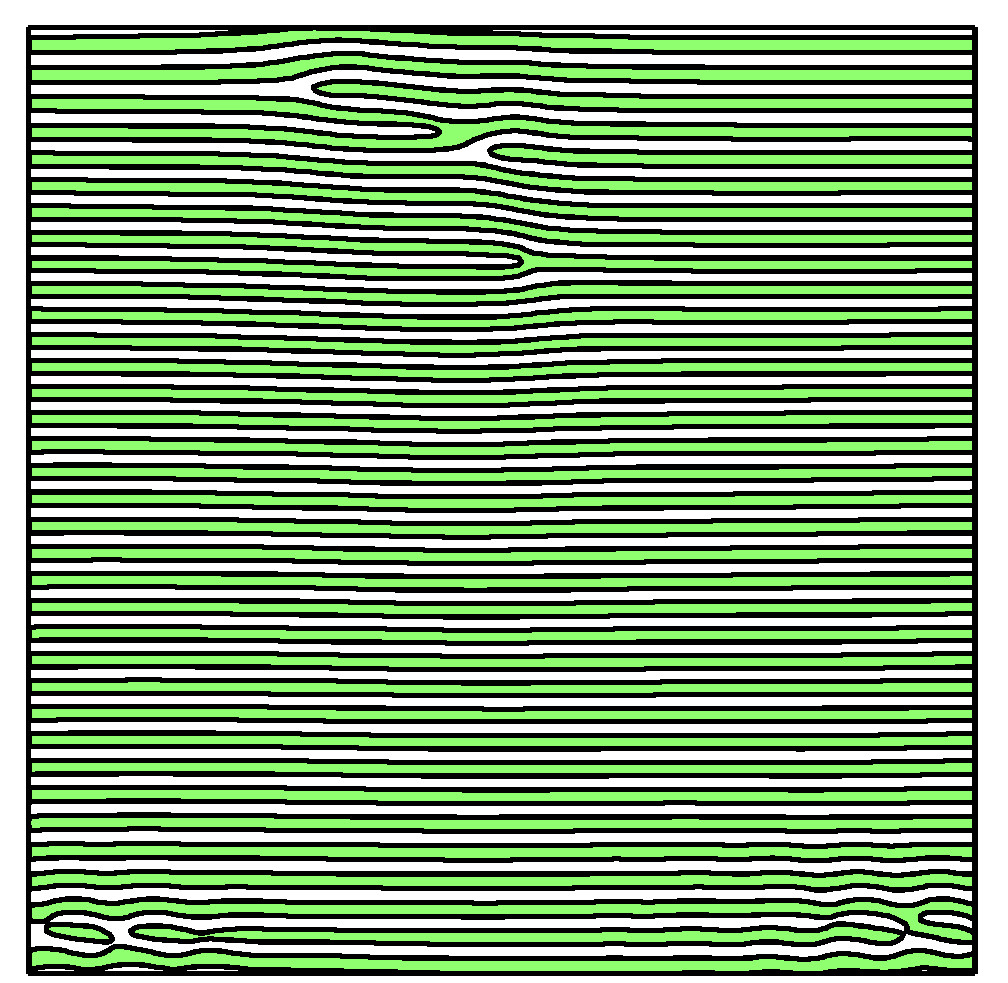
For sufficiently large system sizes, the final steady state is not a perfectly aligned state, but rather a disordered state where there is a dynamical balance between the annealing of defects under shear and the spontaneous creation of defects. Disorder measures are used to quantify the extent of disorder in the system, and these are found to be linked to the rheology. One important observation is the `defect pinning' mechanism, where the region between two edge dislocations moves as a plug with no shearing, resulting in a large apparent viscosity because there is shear in only a part of the domain. The defect dynamics are analysed, and it is found that there is attraction between defects of opposite sign if the are sheared along the compressional axis. The spacing between defects approaches a steady value if the Reynolds number is sufficiently low, but the defects approach and cancel when the Reynolds number is increased. The regions of steady defect spacing and complete annealing of defects are determined as a function of the Reynolds and Schmidt numbers and the dimensinless stiffness. Two different scaling laws are proposed for the approach of defects towards each other and cancellation as the defects are sheared past each other.

The microscopic model based on defect dynamics is used to derive scaling laws for the ordering and viscosity of lamellar mesophases under shear.