(390c) Connection between Local Dynamic Environments & Relaxation Mechanism

Since the 1950s, there has been an interest in deeply exploring transport in amorphous condensed matter phases, but in these seventy years, an in-depth understanding of these phenomena has yet to be attained. As time progresses, however, the importance of the role of β_JG relaxation becomes increasingly apparent; the molecular motion tied to β_JG relaxation is molecules breaking out from their local cage structure by “hopping”. This hopping motion is facilitated by excitations which are local density fluctuations that reduce local energy barriers to motion. In this presentation, I will demonstrate that quantifying these excitations can provide information about the shift in relaxation mechanism of liquids with respect to temperature.

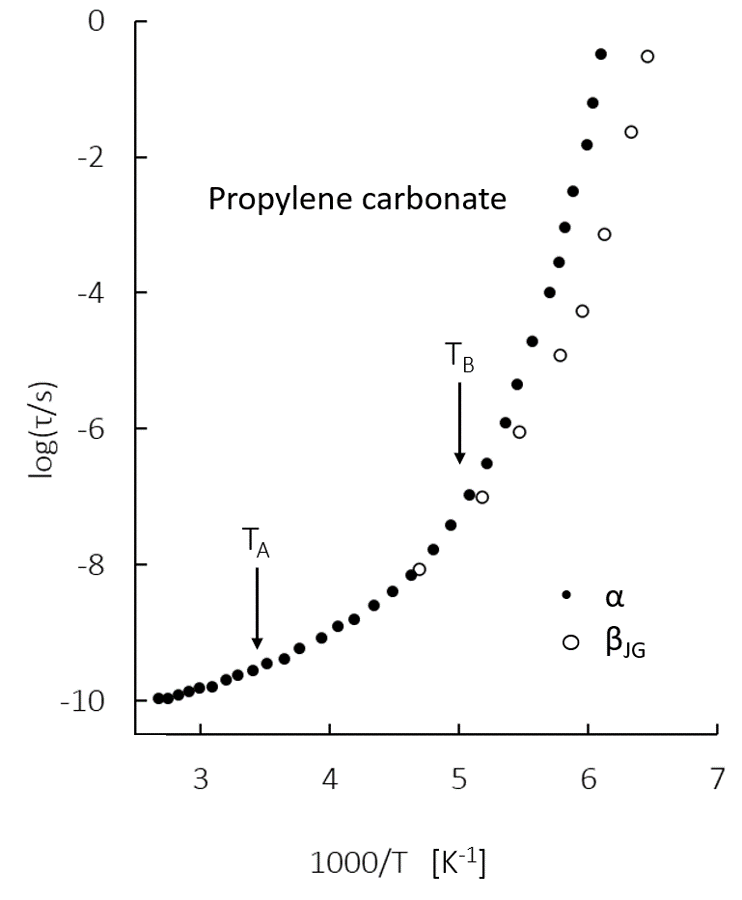
As shown in the figure¹, in the liquid and glass phases, there are temperatures T_A and T_B at which mechanistic changes in relaxation occur². At relatively high temperatures, T > T_A, relaxation is purely collisional and there is no evidence of cooperative motion, while at intermediate temperatures T_B > T > T_A, dynamics follow super-Arrhenius behavior. At low temperatures, T < T_B, a secondary relaxation process that bifurcates from primary relaxation emerges. Using data collected from neutron scattering of five simple liquids³, our group has seen that at T_A and T_B, there is a shift in the average number of excitations in the first shell of the molecule. Further, we can categorize local dynamic environments based on the number of excitations in the first shell; these environments are titled P_iwhere i denotes the number of excitations in the first shell. By assuming a random distribution of these environments, we have seen that at temperatures T_A and T_B, environments P₁ and P_≥2 both have populations of approximately 24%, respectively. These thresholds for excitations paint a fundamental picture of the local processes lead to the mechanistic changes in liquid relaxation. With this information, we reach closer a level of predictive frameworks for liquids comparable to that we have for solids and gases.

1. Schneider et al., Phys Rev E59 (1999)
2. Stickel et al., J Chem Phys 104, 2043 (1996)
3. Cicerone et al., Phys Rev Lett 113, 117801 (2014)